Turing Machines

Turing Machines are the next step in the evolution of automata. They are a theoretical model of computation that can simulate any algorithm.

---

Definitions

Before we get into the mathematical definition, let’s explore what a Turing Machine would look like in the physical world.

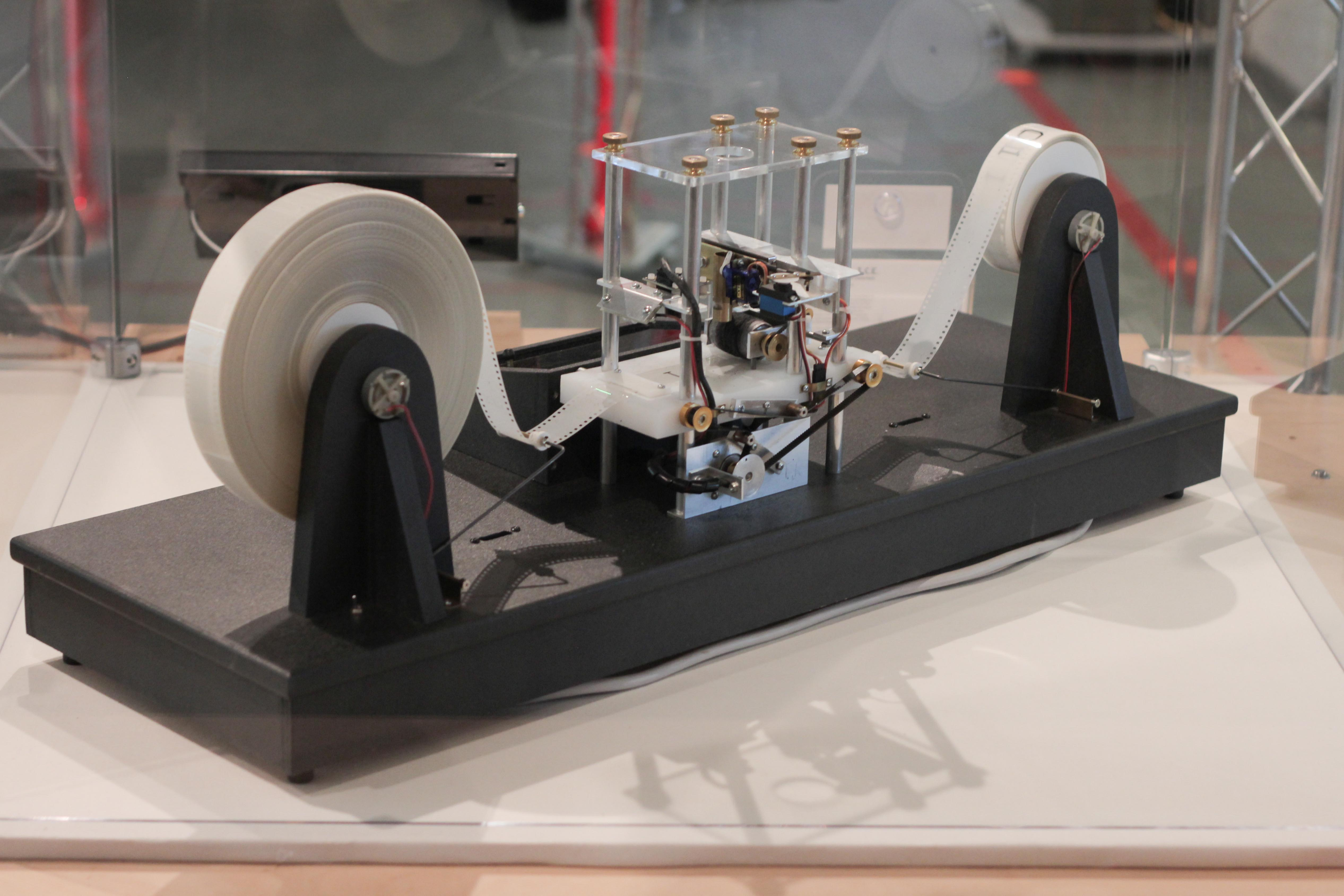
The simple idea of a turing machine is just a tape with a read/write head. The tape is infinite in both directions, and the read/write head can move left or right. The tape is divided into cells, each of which can hold a symbol from a finite set of symbols.

We can define a Turing Machine as a 7-tuple:

$$M=(Q,\Sigma ,\Gamma ,\delta ,q_0,q_{accept},q_{reject})$$

Where:

• Q is a finite set of states
• $\Sigma$ is a finite set of input symbols (input alphabet)
• $\Gamma$ is a finite set of tape symbols (tape alphabet)
• $\delta$ is the transition function
• $q_0$ is the initial state
• $q_{accept}$ is the accept state
• q_{reject} is the reject state

But we already know most of this from previous automata. The new part is the transition function, which is defined as:

$$\delta :Q\times \Gamma \to Q\times \Gamma \times \{L,R\}$$

There are also multiple Turing Machine Variants, but that is for another time.

---

Transition Function in Depth